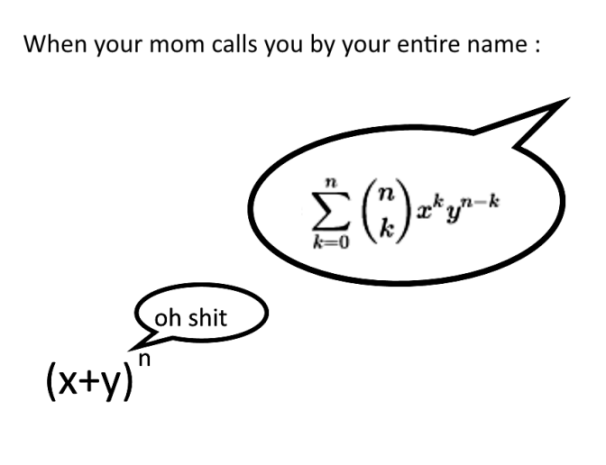
The first two infinite series follow a nice, predictable pattern—the first equals 2, the second equals 1. All is well in math land. Then the third series hits with π²/6 as the answer, and our mathematician's brain short-circuits.
This is the infamous Basel Problem, solved by Euler in 1734. Mathematicians had been banging their heads against walls for decades trying to figure out why this seemingly simple series produces an irrational number involving π squared.
Just another day in mathematics where things make perfect sense until they absolutely, horrifyingly don't. The universe's way of saying "you thought you understood patterns?"

 Academia
Academia
 Ai
Ai
 Astronomy
Astronomy
 Biology
Biology
 Chemistry
Chemistry
 Climate
Climate
 Conspiracy
Conspiracy
 Earth-science
Earth-science
 Engineering
Engineering
 Evolution
Evolution
 Geology
Geology