Contradiction Memes

That moment when you're driving the bus of mathematical creativity and someone points out your number system violates the fundamental laws of algebra. The mathematical equivalent of being pulled over for breaking the laws of physics. Every mathematician has that "j = √(-1)" phase where they think they've revolutionized math, only to discover that 1 = 2 and reality implodes. The universe's way of saying "nice try, but I prefer consistent arithmetic."

The mathematical hypocrisy is real! Mathematicians will lecture you about how dy/dx isn't technically a fraction, then turn around and write it as \frac{dy}{dx} in LaTeX, making it look exactly like a fraction. This is the equivalent of saying "I'm not eating cake" while literally shoving cake in your mouth. The notation contradiction perfectly captures that special brand of mathematical doublethink where formal definitions and practical usage are in constant tension. And yet we all just accept this bizarre contradiction because... math gonna math. Next they'll tell us imaginary numbers aren't actually imaginary while writing "i" everywhere.

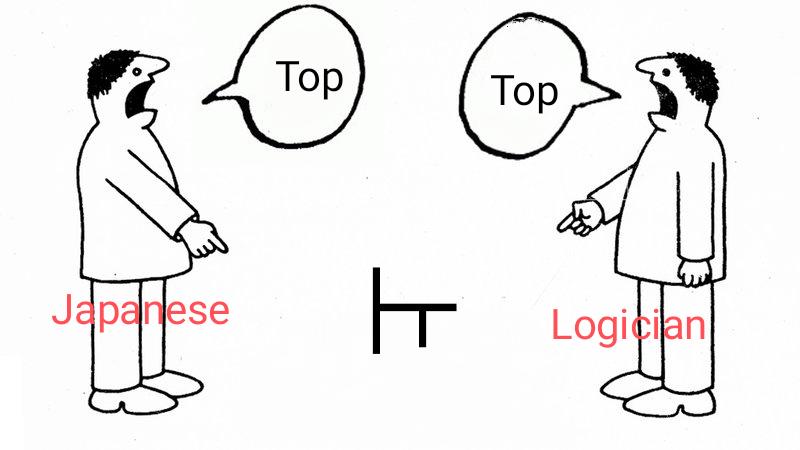
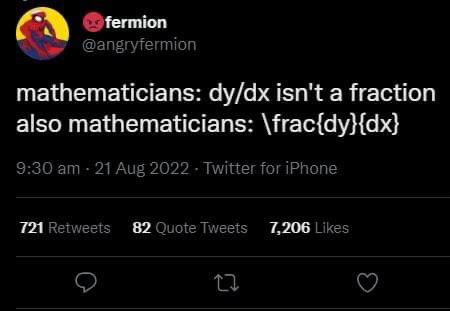
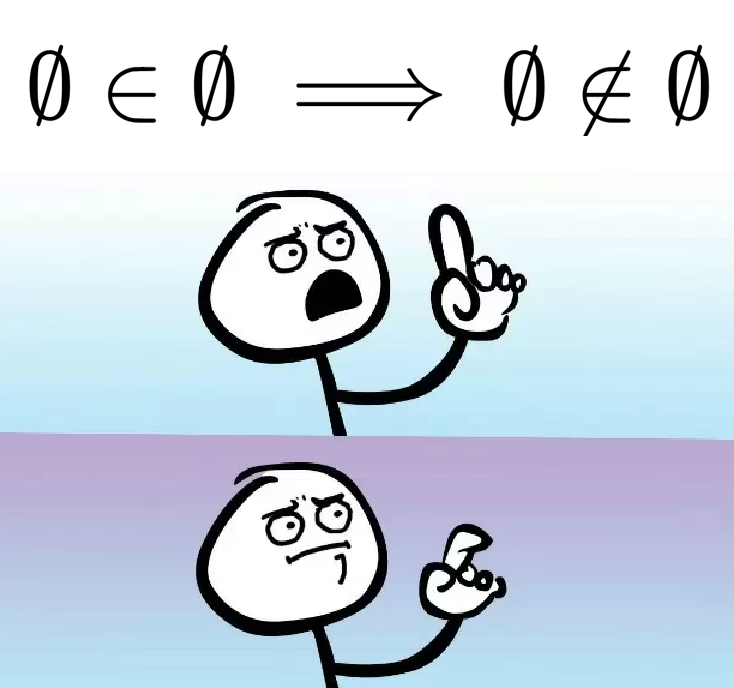The mathematical debate between Pokémon characters perfectly captures the chaos of factorial notation! In the first panel, the green character states "2!=2" which is mathematically correct (2 factorial equals 2), earning approval from the blue character. But when claiming "0!=1" in the second panel, both characters respond with hearts despite the green one previously rejecting it as wrong. Plot twist: 0! actually DOES equal 1 by mathematical definition! It's that rare moment when the seemingly absurd answer is correct, just like when your wildest hypothesis turns out to be true. The factorial function contains multitudes of surprises, much like these adorable creatures contain multitudes of opinions.

Nothing like a good mathematical contradiction to break a student's spirit! The meme shows the empty set supposedly being an element of itself (∅∈∅), which implies the empty set is not an element of itself (∅∉∅). Pure mathematical chaos. For the uninitiated, the empty set contains absolutely nothing – not even itself. Trying to claim it contains itself creates a paradox that would make Russell and his barber-who-shaves-himself puzzle look like child's play. The stick figure's journey from shocked realization to defeated acceptance perfectly captures every math major's existential crisis. First-year students think math is about numbers. By graduation, they realize it's about crying over symbols that shouldn't logically exist.

The mathematical cold war continues. Constructivists believe you should directly build mathematical objects to prove they exist, while contradiction fans just assume something doesn't exist and wait for reality to implode. It's like telling a constructivist "I proved my keys exist by assuming they don't and then finding a contradiction when I drove to work." That fist in the air isn't celebration—it's the universal symbol for "I will construct a counterexample to your entire existence."

Linear algebra without matrices is like trying to swim without water. The mathematical equivalent of ordering a cheeseburger, hold the cheese. Somewhere, a mathematician is having a nervous breakdown looking at this book. Next up in the series: "Calculus Without Derivatives" and "Geometry Without Shapes." Graduate-level masochism at its finest.

Imagine Gottlob Frege, the father of modern logic, absolutely losing it over a mathematical paradox! The meme shows him raging about Russell's Paradox - that mind-bending set R = {x | x ∉ x} that broke set theory. For the uninitiated: this set basically asks "Does the set of all sets that don't contain themselves contain itself?" If it does, then it doesn't. If it doesn't, then it does. 🤯 Poor Frege was about to publish his life's work when Russell sent him this paradox, essentially saying "Hey buddy, your entire mathematical foundation has a tiny problem - it's completely inconsistent." Talk about a bad day at the philosophy office!

That moment when your brain decides 2:39 AM is the perfect time to ponder mathematical impossibilities! The meme highlights a classic mathematical contradiction: if π = e (which they absolutely don't), then their squares would equal 9 and 10 simultaneously. In reality, π ≈ 3.14159... and e ≈ 2.71828..., making this mathematical nightmare fuel. It's basically the equivalent of your brain forcing you to divide by zero when all you want is sleep. Mathematicians everywhere just felt a collective shudder.