Torus Memes

The ultimate mathematical dad joke! What you're seeing is a genus-2 surface (a double torus) that looks suspiciously like a pair of jeans. In topology, we don't care about exact measurements—only the fundamental shape properties. So to a topologist, your fancy designer jeans and this mathematical monstrosity are essentially identical. While Sydney Sweeney might break the internet with her denim, topologists break mathematical conventions with surfaces that have exactly the right number of holes. Fashion is temporary, but topological invariants are forever.

The perfect collision of abstract math and real life! The top panel shows topological equivalence - where mathematicians consider a donut and a coffee mug to be identical shapes because they both have exactly one hole. In topology, it's not about appearance but the fundamental properties that remain unchanged during continuous deformation. Then reality strikes! The bottom panel shows someone trying to unlock a bike with a combination lock - suddenly topology becomes VERY relevant. Try explaining to your stolen bike that "technically" your lock was topologically sound! Turns out mathematicians' casual dismissal of practical geometry might not hold up when your transportation depends on it. Pure math meets street smarts in the most painful way possible!

The experiment was going smoothly until the coffee mug showed up! What we're witnessing is a topologist's nightmare - three perfect toruses (donuts) in a row and then BAM! A simple coffee mug crashes the topology party! In the wild world of topology, a coffee mug and a donut are actually the same shape (both have exactly one hole), but try telling that to the scientist monitoring this experiment! The stick figure's "all good so far" comment is about to age like milk left in a quantum physics lab over spring break. That mug is the mathematical equivalent of wearing socks with sandals to a fashion show!

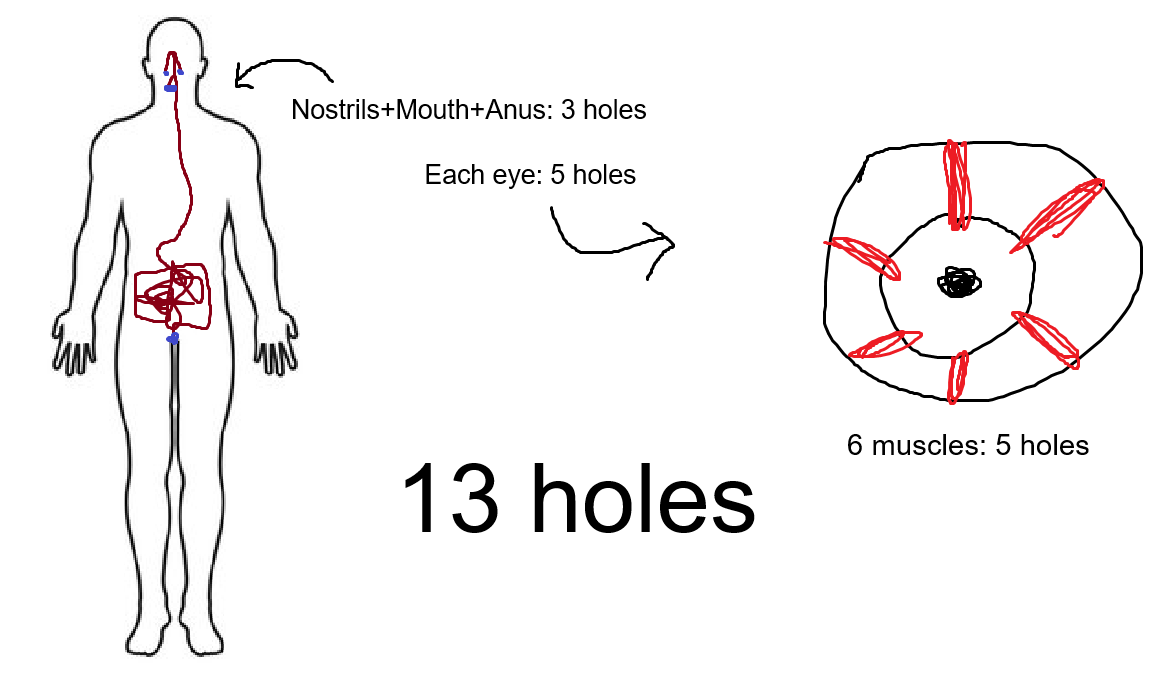
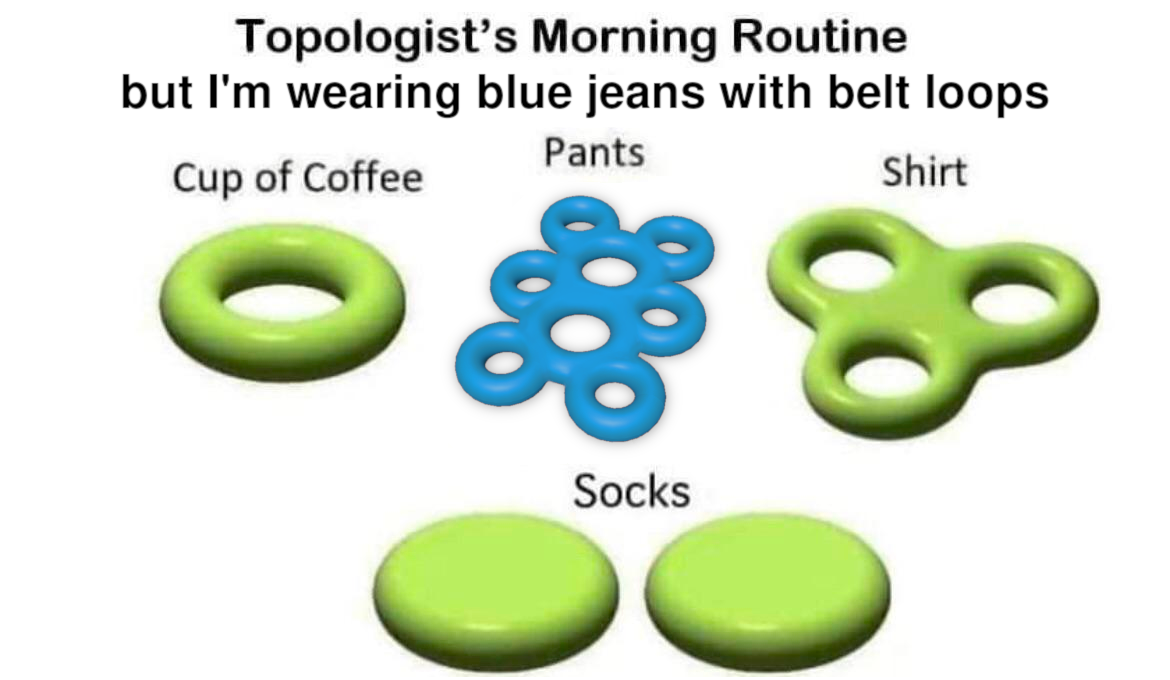
Topologically speaking, your body is just a fancy donut with 13 holes! The digestive tract creates one continuous tunnel from mouth to... exit, making us technically a torus. Add in the tear ducts, nostrils, and other biological plumbing, and congratulations—you're basically walking Swiss cheese according to mathematicians. Next time someone calls you "well-rounded," just tell them it's your genus number talking! For the uninitiated, in topology (the mathematical study of shapes), a donut and a coffee mug are identical because they both have exactly one hole. The "genus" is just fancy math-speak for "how many holes does this shape have?" So humans having genus 13 means we're basically the fanciest, most complicated donut at the bakery!

To a topologist, a coffee mug and a donut are identical—they both have exactly one hole. This meme takes that concept to your wardrobe! The coffee cup is a simple torus, the shirt has three holes (one big one and two arm holes), and the socks are just spheres (zero holes). But those pants? That's where the joke gets its punch. Those aren't regular pants—they're "blue jeans with belt loops," meaning they're topologically distinct with multiple holes. In topology, it's not shape that matters but the number of holes. Your fashion sense might be questionable, but your topological classification is impeccable!

The mathematical equivalent of finding out your spelunking gear doesn't fit! This meme brilliantly pokes fun at topology, where a worm (or mathematician) is contemplating exploring what appears to be a horn torus or funnel shape. The title refers to the holes in the letters Q and R - because in topology, these letters have fundamentally different structures (Q has one hole, R has two). It's basically what happens when mathematicians try adventure sports - they get stuck analyzing the genus of the cave instead of actually exploring it. Next paper title: "On the Impossibility of Fitting Through an ε-Sized Opening."

Dating in topology is rough. Left: you (a simple torus/donut shape with one hole). Right: the guy she tells you not to worry about (a Klein bottle with non-orientable surface and zero boundaries). Sure, you both have genus 1, but he's got that exotic self-intersecting structure that defies 3D space. Mathematicians call this getting "non-orientably outclassed." At least you're embeddable in regular 3D space without cheating.

Mathematicians have a unique relationship with mugs. Normal people buy those pretentious formula-covered mugs thinking they look smart, while real math nerds just want a donut mug because... well... topology . In topology, a coffee mug and a donut are actually the same shape (homeomorphic) - they both have exactly one hole! So that donut "mug" is the ultimate inside joke. The final panel is just the mathematician sitting there smugly thinking "I'm not drinking from a mug, I'm drinking from a torus." Pure mathematical superiority in ceramic form.

Behold the mind-bending world of topology, where mathematicians ignore normal geometry and focus on properties that don't change when objects are stretched or twisted! In this hilarious brainteaser, we see a cigarette poking through different holes of a torus-like shape, making us question which way a "topological human" would actually smoke. Because in topology, it's not about the specific location—it's about the connectivity! The cigarette could go through ANY hole and still be mathematically equivalent. It's like saying your coffee mug is technically the same as a donut. (Your morning routine just got way more confusing!)

The mathematical torus is having an existential crisis! While it's a superstar in topology (the branch of math studying shapes that remain unchanged under stretching and bending), it can't help comparing itself to its tastier look-alikes. Poor torus—geometrically fascinating with its donut shape and one hole, yet forever walking past regular donuts and bagels with food envy! In topology, a torus is basically a surface with one hole—like a donut where mathematicians care about its hole properties, not its deliciousness properties. The ultimate shape-identity crisis!