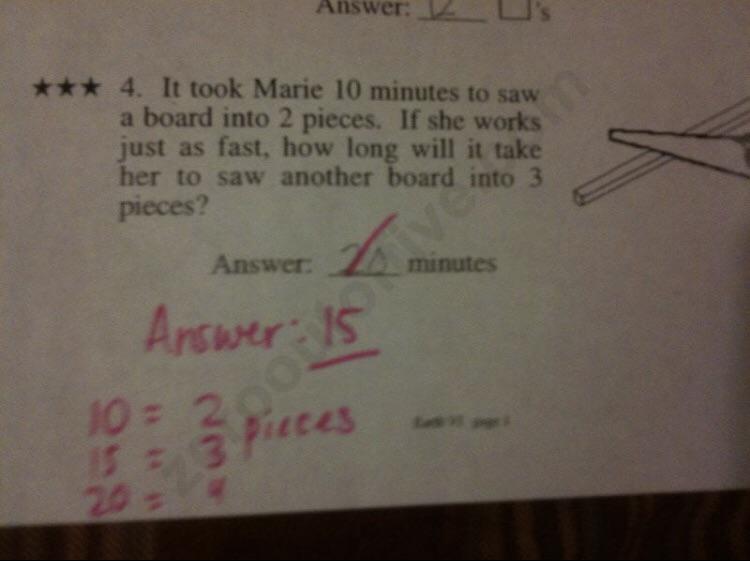Proportional reasoning Memes

The student's answer is a beautiful demonstration of linear thinking in a non-linear world! They've assumed that if 10 minutes = 2 pieces, then 15 minutes = 3 pieces. But they missed the crucial detail—cutting a board into 2 pieces requires ONE cut, while cutting it into 3 pieces requires TWO cuts! This is basically the mathematical equivalent of thinking you can cook two chickens in the same time as one chicken. The correct answer is 20 minutes (2 cuts × 10 minutes per cut). Math teachers everywhere are silently screaming into their coffee mugs right now.

The eternal battle between linear and non-linear thinking! The teacher expects the answer to be 20 minutes (assuming 10 min per cut), but our green monster student realizes it's actually 15 minutes. Why? Because Marie needs 2 cuts to make 3 pieces, not 3 cuts! It's a classic rate problem that trips up even seasoned problem-solvers. The key insight: count the cuts, not the pieces. For n pieces, you need (n-1) cuts. The student's logic is flawless - if 10 minutes = 1 cut (creating 2 pieces), then 2 cuts (creating 3 pieces) would take 20 minutes. But wait! The original problem stated 10 minutes for the WHOLE JOB of creating 2 pieces, not per cut! This is why engineers triple-check their assumptions before building bridges. One wrong assumption and suddenly your Mars orbiter is playing hide-and-seek with the Martian surface!